Tool/software: WEBENCH® Design Tools
I'm trying to design to a a bandpass filter with the filter design tool and I'm struggling to understand a few things from the design. The design parameteres required is a filter with a:
Bandwidth: 60KHz
Centre Frequency: 130 kHz
Gain: 40dB
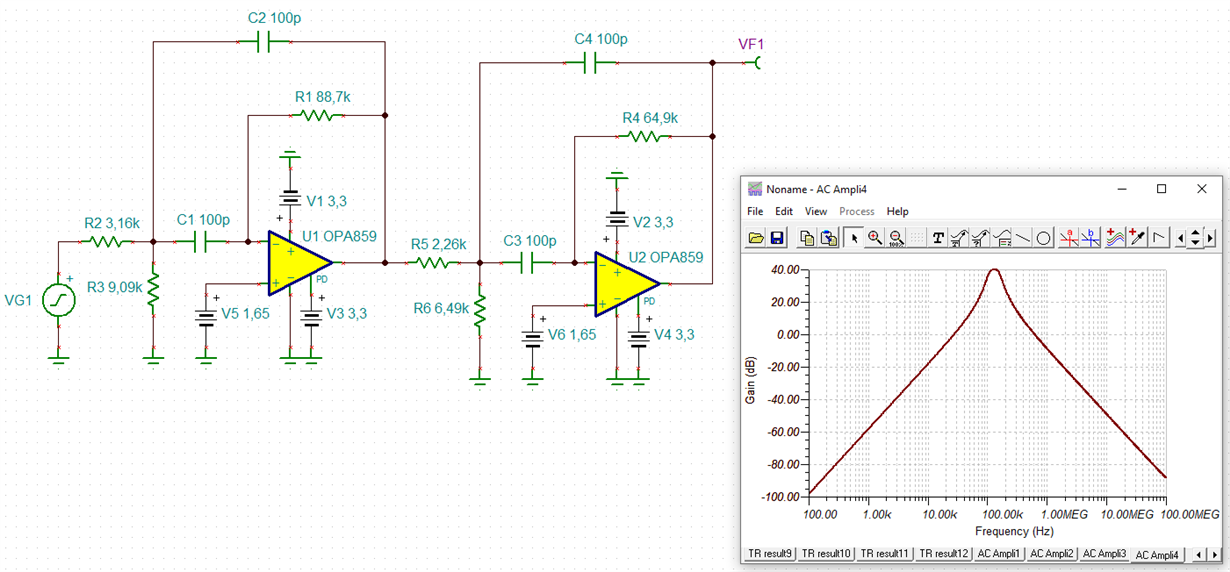
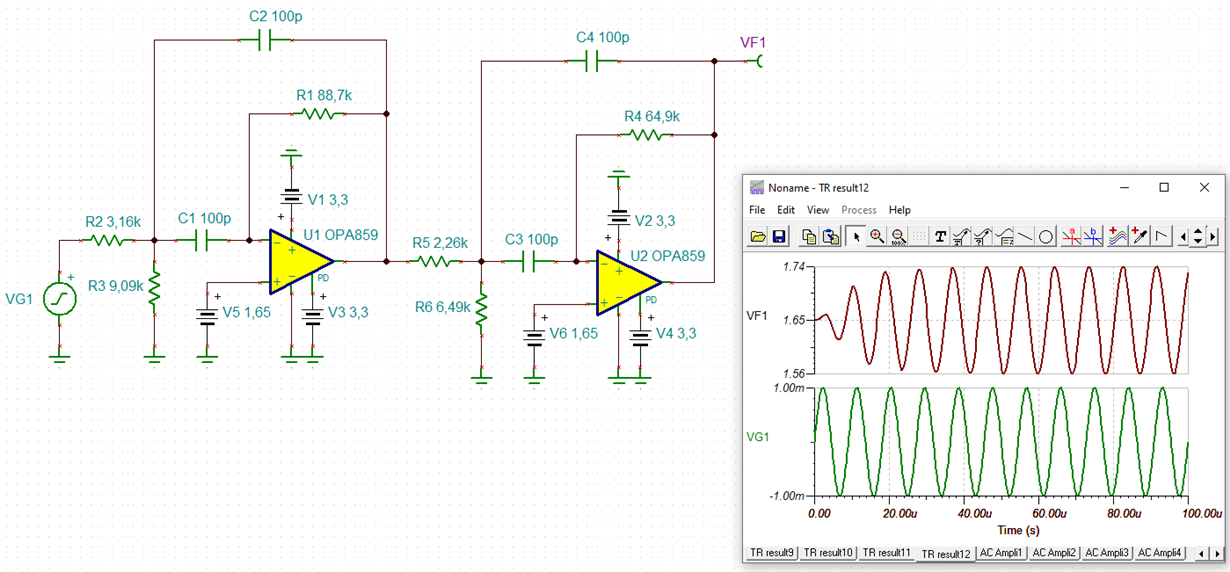
I also wanted an amplifier that goes with Vmin of 0V and VMax of 3.3V so I entered these values in the design section as well. The tool suggested the OPA859 and the following circuit was suggested:
But looking at the summary, the minimum gain bandwidth required at each stage just looks absurdly large to me:
Filter stage 1 is given as:
Cutoff Frequency 110.357 kHz
Min GBW Reqd 490.91 MHz
Stage Gain 14.035 V/V
Stage Q 3.075 Stage
Topology Multiple Feedback
Stage 2 is given as:
Cutoff Frequency 152.59 kHz
Min GBW Reqd 682.279 MHz
Stage Gain 14.358 V/V
Stage Q 3.111
Stage Topology Multiple Feedback
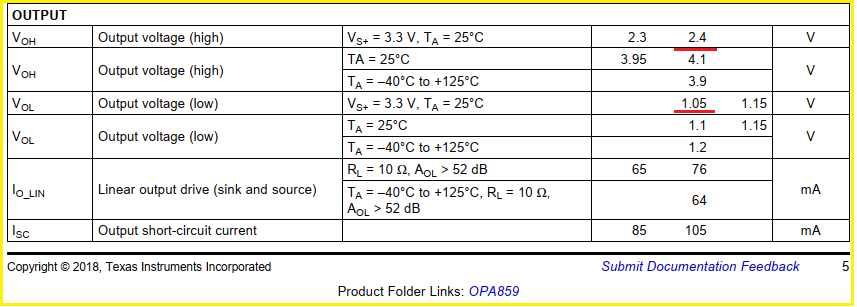
Additionally, it's not quite clear where I bias the circuit. If I want a midpoint of 1.65V, Shouldn't I simply connect 3.3V to Vcc, 0 to Vee, then 1.65V to both positive inputs of the op-amp and the R3_S1/R3_S2 ? I appreciate any clarification,
thanks.