Hi TI support team,
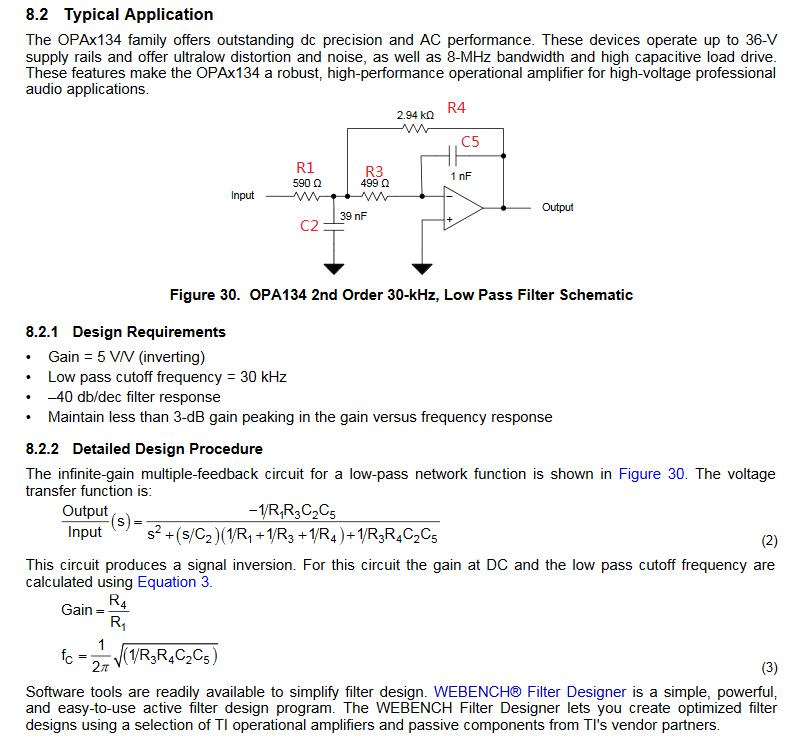
I have a question on page 16 of the OPA134 datasheet. In the typical application, the low pass cutoff frequency is 30 kHz, but my calculation based on Formula (3) is about 21 kHz. I have simulated this circuit on TINA and the filter's - 3dB frequency is about 30kHz. I am confused about these two results.