Did you miss last week’s brain-teaser? Go back if you’d like to test your skills before seeing the answer below.
Repeating the question… what is the resistance of this infinite resistor network?
Though this can be solved numerically (and very accurately) there is a closed-form solution and I challenged readers to submit an answer with an explanation. The key is in realizing that second resistor is in parallel with the remaining infinite network which still has a value of R.
I received answers from three readers. The first, from Sarmad Nasir in California, arrived just a few hours after the puzzle was posted. He called the total resistance Req and provided this description:
The 1 Ohm resistor is represented by R is my closed-form expression and the total equivalent resistance is called Req:
I replaced all the resistors to the right of the leftmost two with a lumped resistance Req. I can do this because it is an infinite network and the equivalent resistance should be the same even after I consider the leftmost two resistors. The lumped resistance Req is in paralleled with the vertical 1 Ohm resistance, and together, this is in series with the first horizontal 1 Ohm resistance. The total resistance of these three resistances still equals Req. Solving for Req then results in the closed-form expression above.
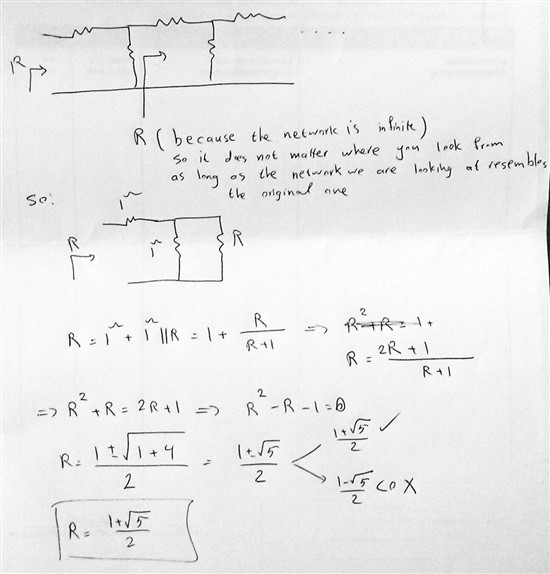
And I appreciated the hand-written solution below from Behnam Molavi in Vancouver, BC, showing results from the quadratic formula:
I must also mention a correct solution from Alejandro Gamero with warm greetings from Chile. Thanks, Alejandro.
Thanks to you three for submitting solutions. I bet there were many others who solved the puzzle but did not send an answer. I hope that you found the exercise satisfying.
Next week The Signal is back to work with a discussion on op amp noise. Happy New Year and thanks for reading. Comments are welcome.
Bruce email: thesignal@list.ti.com (Email for direct communications.)
Table of Contents for all The Signal blogs.