Other Parts Discussed in Thread: TINA-TI
Hi all,
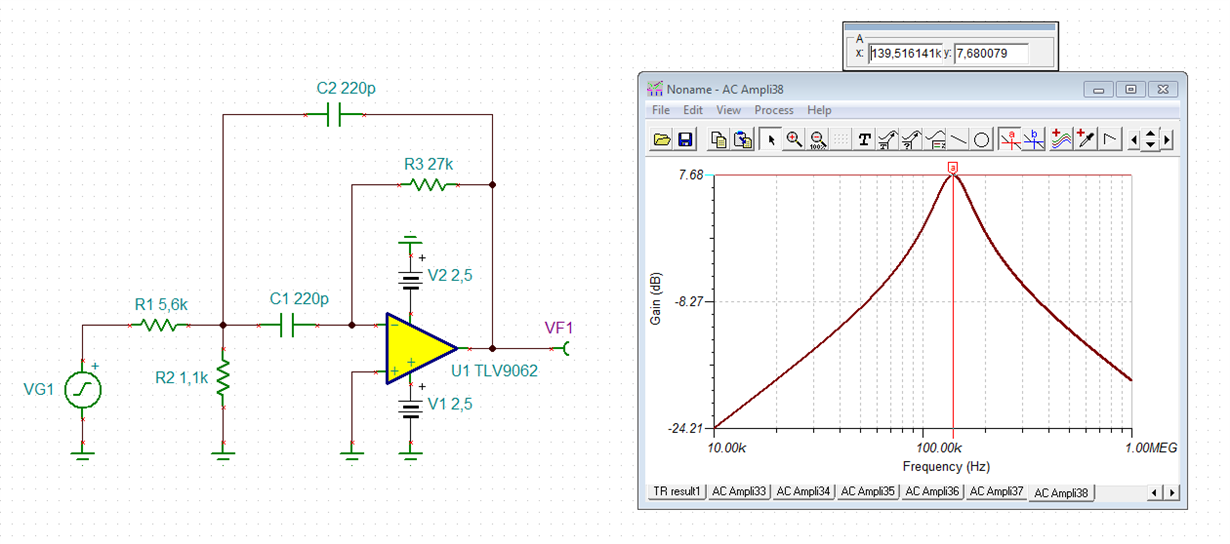
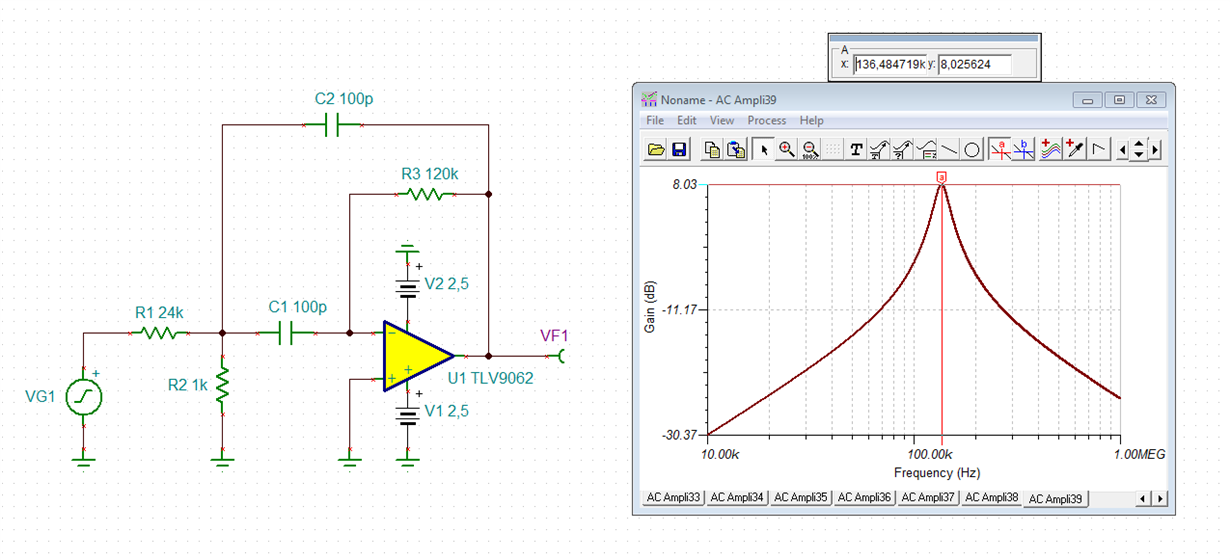
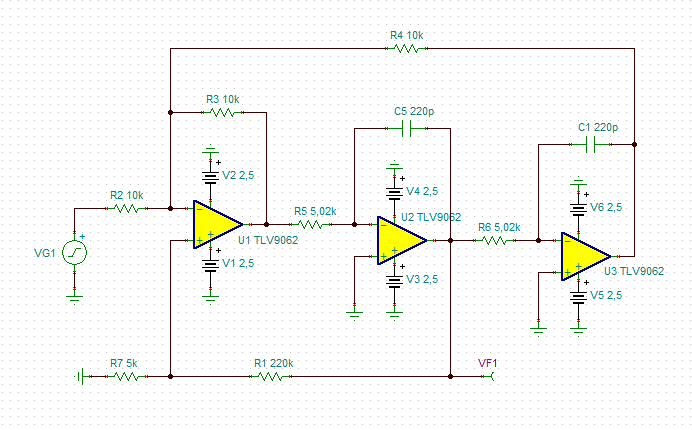
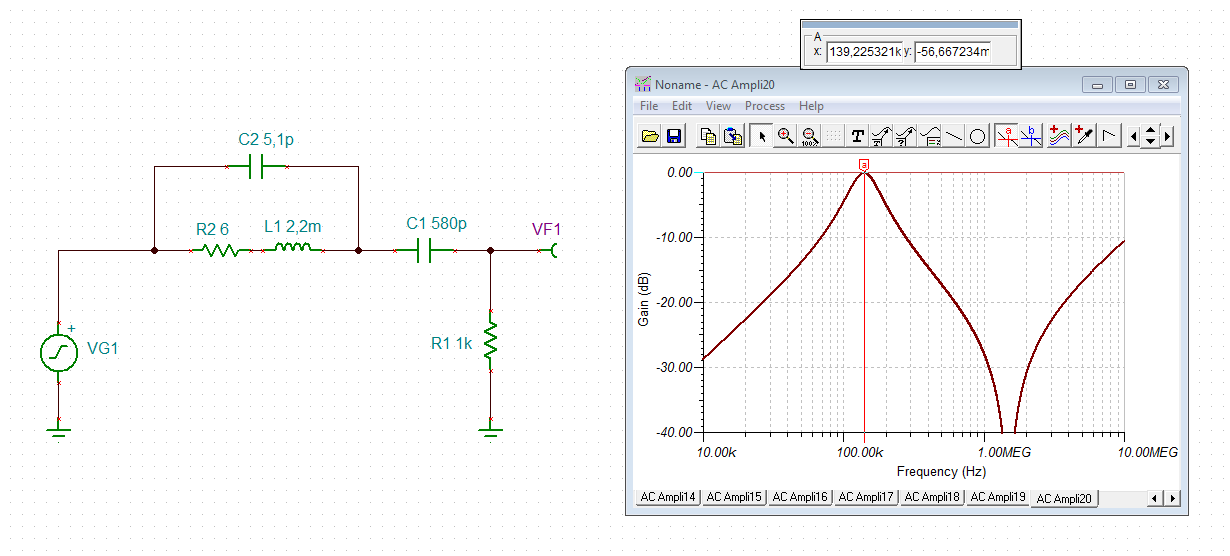
I'm using a TLV9064 quad op-amp in a cascaded active bandpass filter configuration. I fine-tuned my design using simulations and all is working fine now. But despite the 10MHz GBW of this op-amp the center frequency of my band-pass filter is still slightly dependent on variations in GBW.
The datasheet only mentions a typical value for GBW. Is there any information available about the variation in GBW? Does it also change with temperature?
Thanks.
Paul
--