Hi TI Engineers!
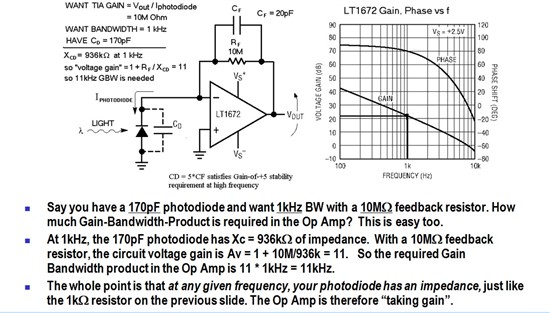
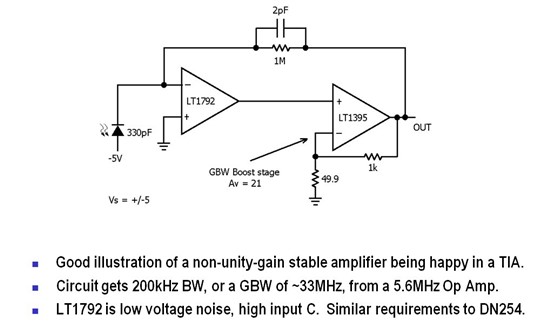
I had read an article about transipedance amplifier (TIA) that explains how to figure out the TIA's GBW as shown below.
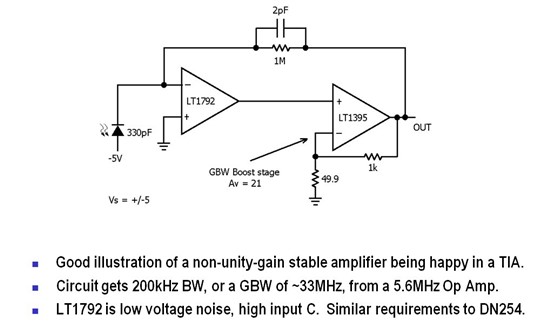
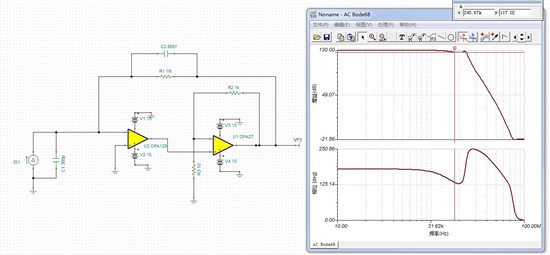
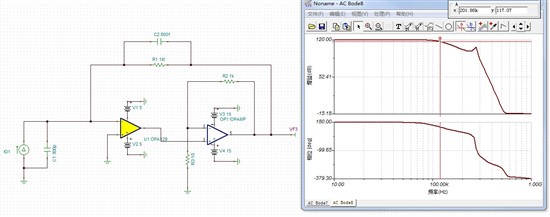
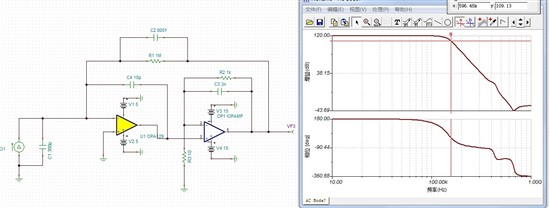
My first question is :does this explaination useful in an composite circuit ? Bescuse the article gives a circuit desgin "Speed up a JFET-INPUT OP AMP " as shown below. The GBW is 33MHz ,which does not equal to Gain(471)*Bandwidth(200KHz)=83.4MHz. The Gain 471 is from 1+1M / X330pF=1+1M / 2.4K=471. So I confused.
The next question is : In this composite circuit , what is the relationship between the Av of Boost stage and the whole circuit GBW? How can I choose other op amp to replace the LT1792 and LT1395? Can OPA 635 be the first op amp to replace LT1792 and op27 be the second op amp to replace LT1395?
Thanks!
Best Regerds!
Eva