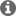Other Parts Discussed in Thread: OPA320, LMP7717
I need something close to a 1 Mhz TIA for interfacing to a photodiode. It has to work indoors and outdoors, so I'm looking at DC coupled, but including an additional op amp that will keep the TIA biased regardless of DC (or low frequency) noise:
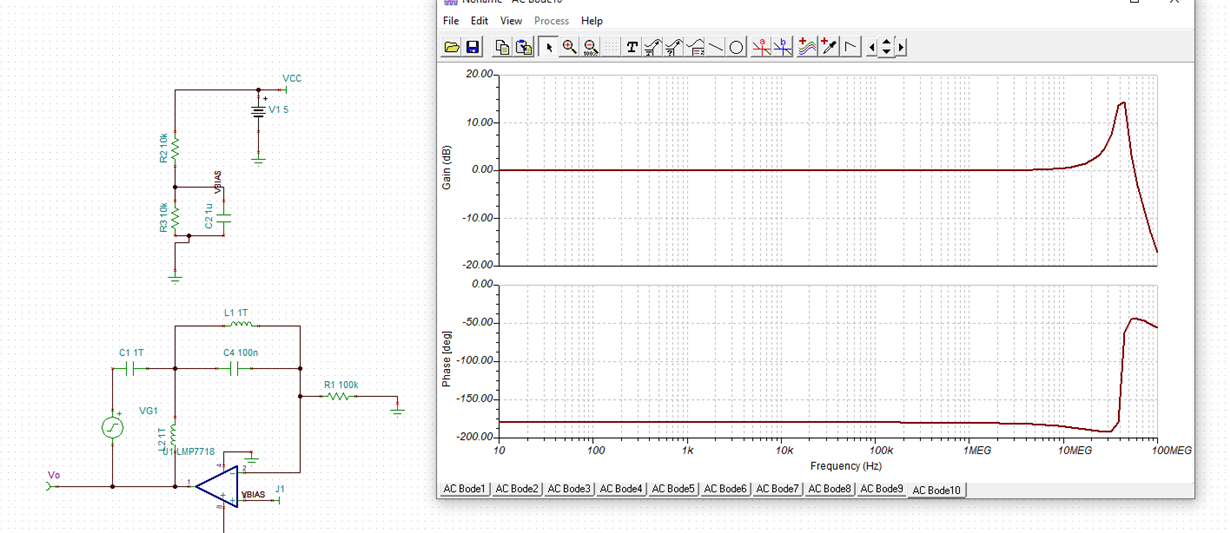
(The 1T inductor and capacitor are just there to break the loop for AC analysis).
The design will need 24 of these (pair of light curtains), so op amp cost is important. Using an uncompensated op amp like a LMP7718 seems more cost effective than using the OPA320. But, I suspect that I need additional stability compensation.
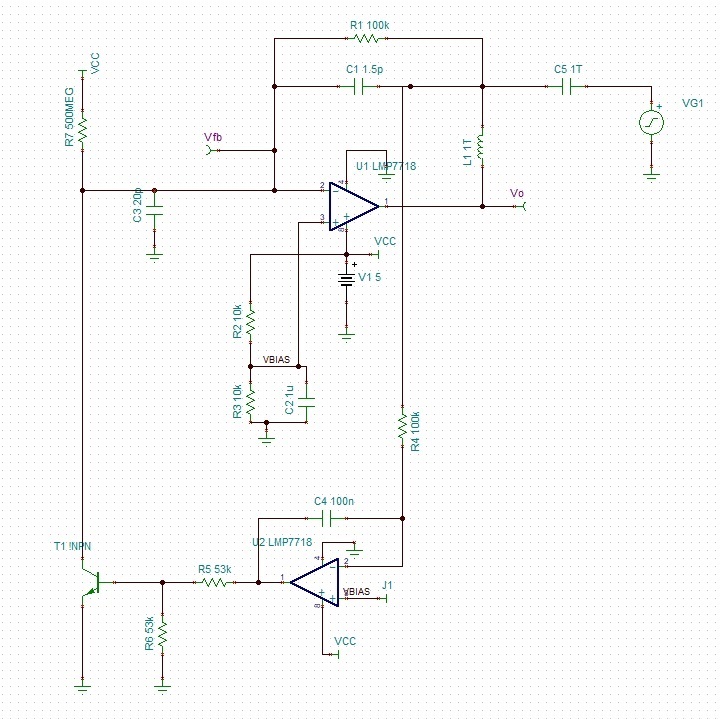
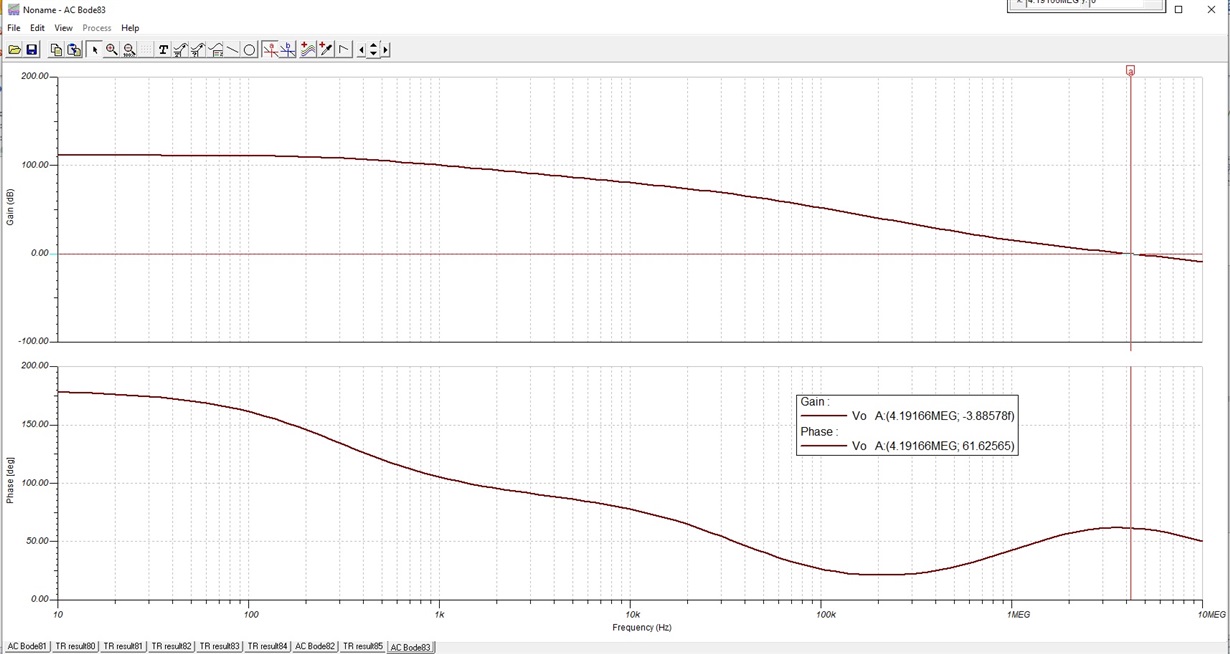
When I do the open loop plots, I get this:
It's been a long time since I did this type of a thing for a living, so please forgive me if I'm asking a stupid question.
Literally, the phase margin is 61 degrees--at least the phase of open loop gain when the gain is zero. But, notice the phase got much closer to zero (41 degrees) when the gain was still quite high. Don't I have to worry about that?
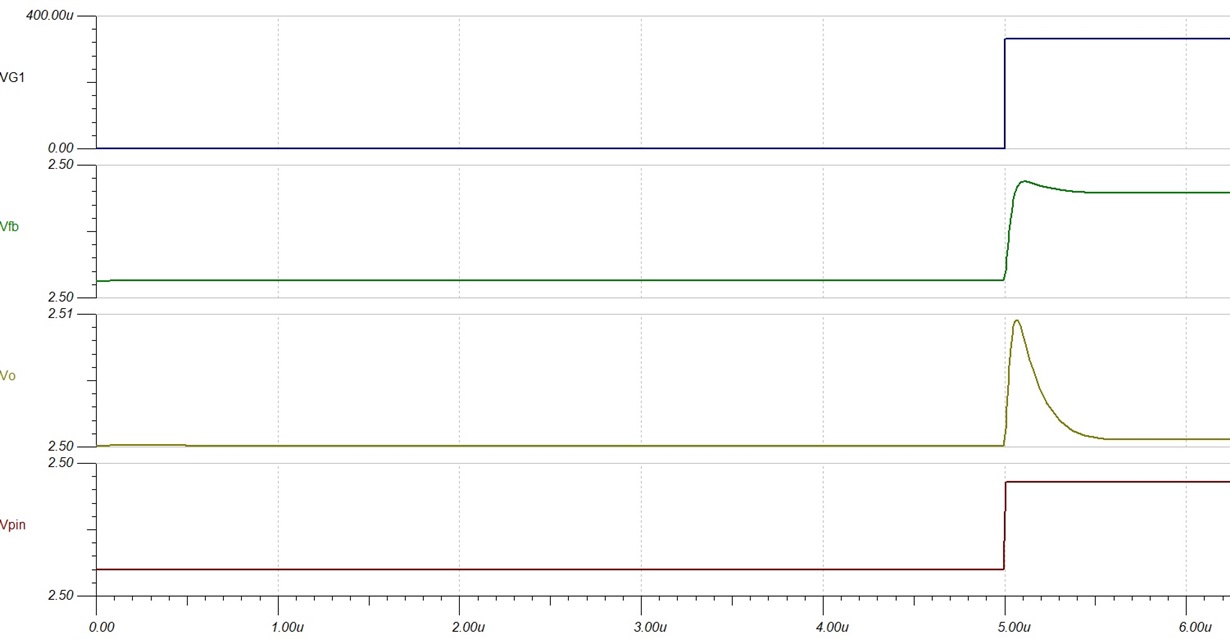
I did a step response and saw a very large amount of peaking. Several times bigger than the settled output change.
Am I correct in thinking I've got a stability problem? Changing the feedback cap doesn't seem to affect the phase margin where the gain is still very positive, nor the frequency at which that occurs. Changing the cap in the DC feedback amp doesn't change things much either.
This is an uncompensated op amp, but I saw very similar results with the OPA320. Everything made sense if I used AC coupled instead of DC coupled with the DC servo. But, when I add the extra op amp generated feedback, I'm getting lost.
Any help would be appreciated!