Other Parts Discussed in Thread: TINA-TI, OPA858, OPA340
Hallo together,
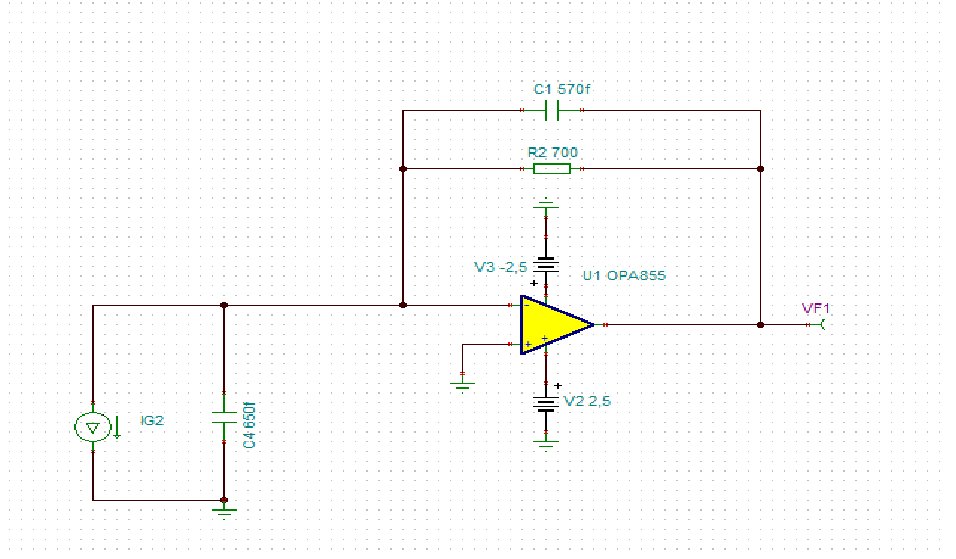
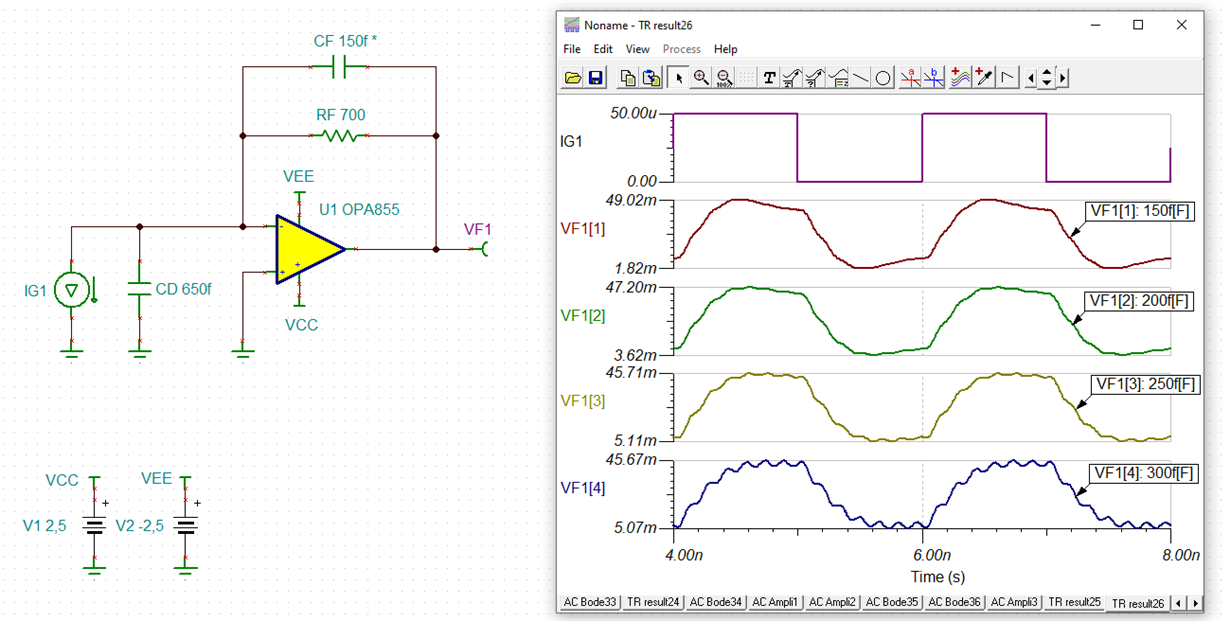
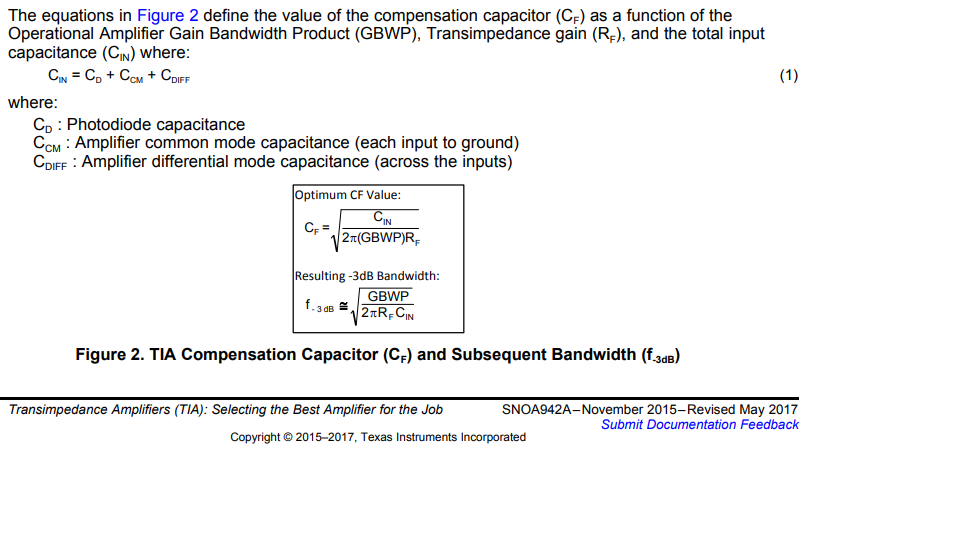
I have a few questions regarding the TIA circuit with OPA855. I have simulated OPA 855. The desired 3dB bandwidth is 400 MHz and needed gain is 700 Ohm.
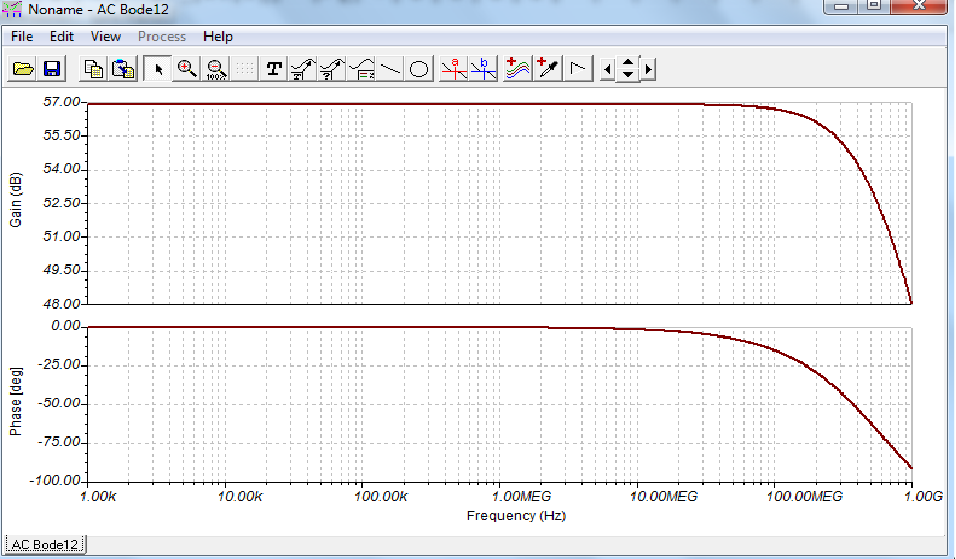
Below you can see my circuit , gain and phase response.
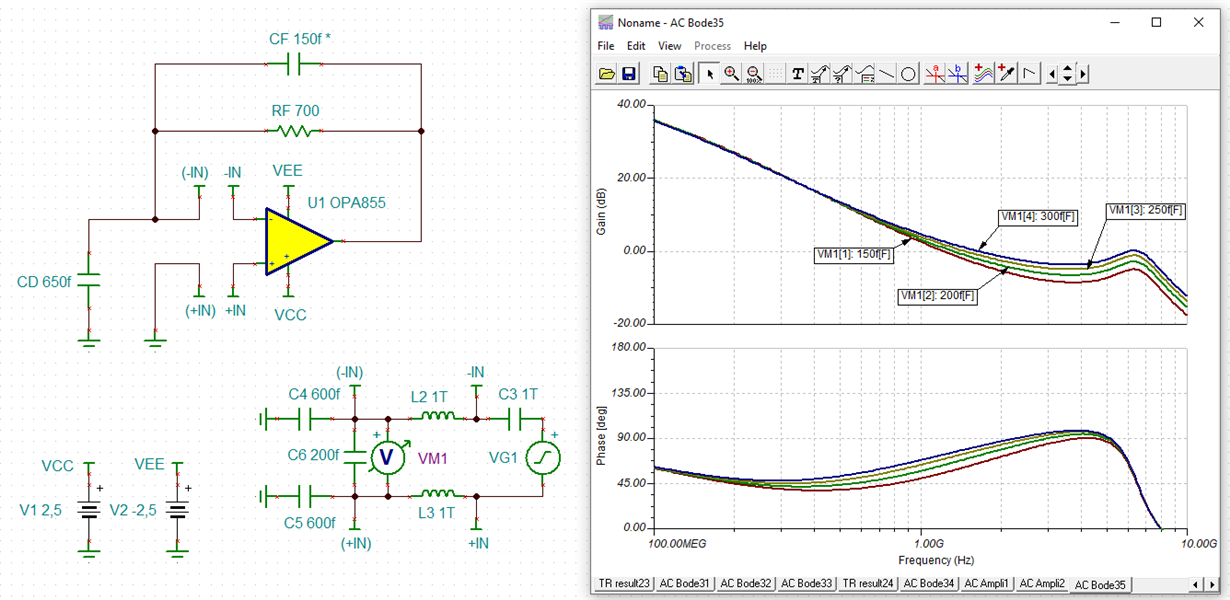
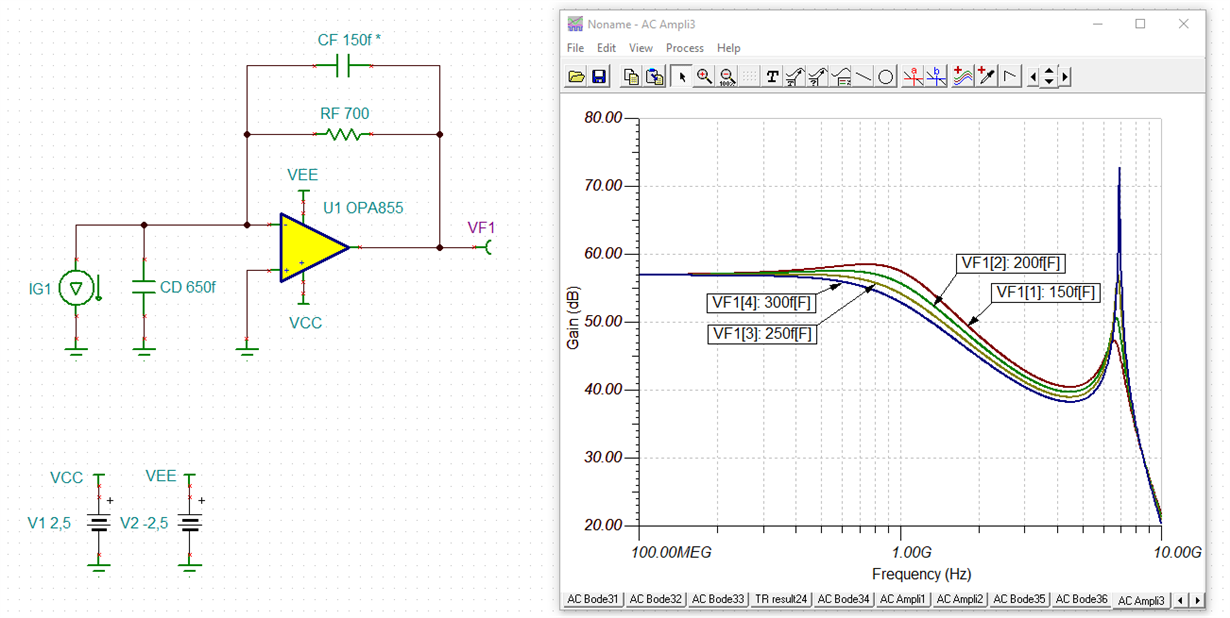
Is the loop gain shown in the picture below? I mean AOL curve + noise gain curve or ? How can I draw this curve separately in TINA?
Then at the intersection of AOL and noise gain curve I can read the phase margin. Now how can I know if this circuit is stable and why is phase negative?
I know that the circuit is stable when the phase margin > 45 degrees.
Thanks,
Selvedin