Hi there,
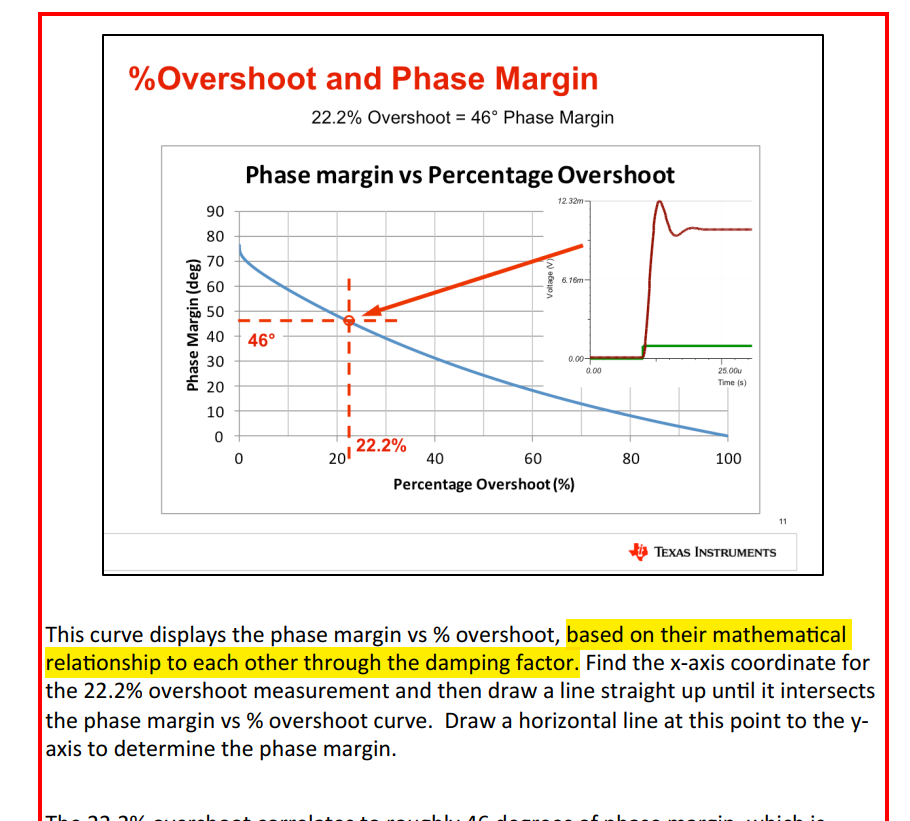
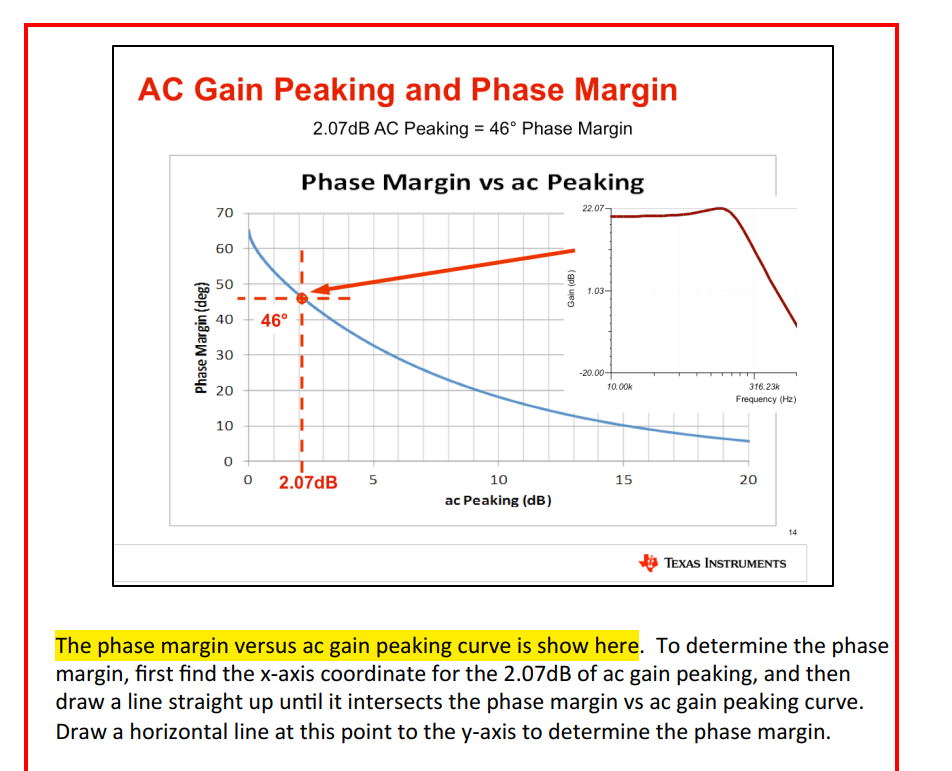
I am learning Precision Labs 10.4 - Op Amps: Stability 4, and I am confused how can user get the curves between phase margin and overshoot % and AC gain peaking on page 11 and 16 of the PPT slide, as below. Seems there is more detailed on about these two curves in the video.
Appreciate some expert could help advice. Thanks.
Address of the slide for the video : training.ti.com/.../1334 Stability 4 - slides.pdf