Other Parts Discussed in Thread: TINA-TI, OPA334, OPA375, OPA344, OPA2375, TLE2426
Tool/software:
Hello,
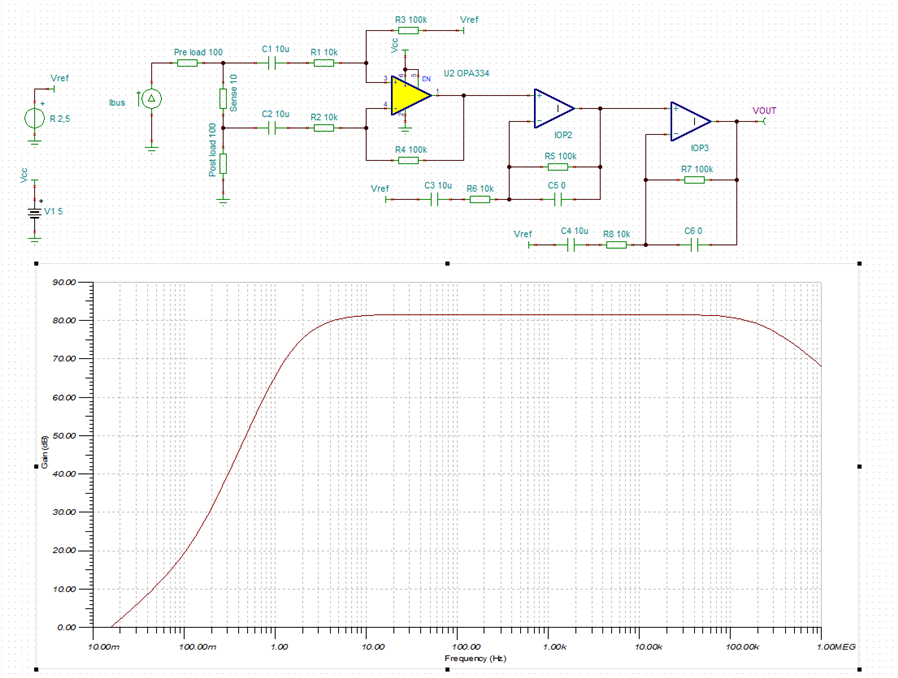
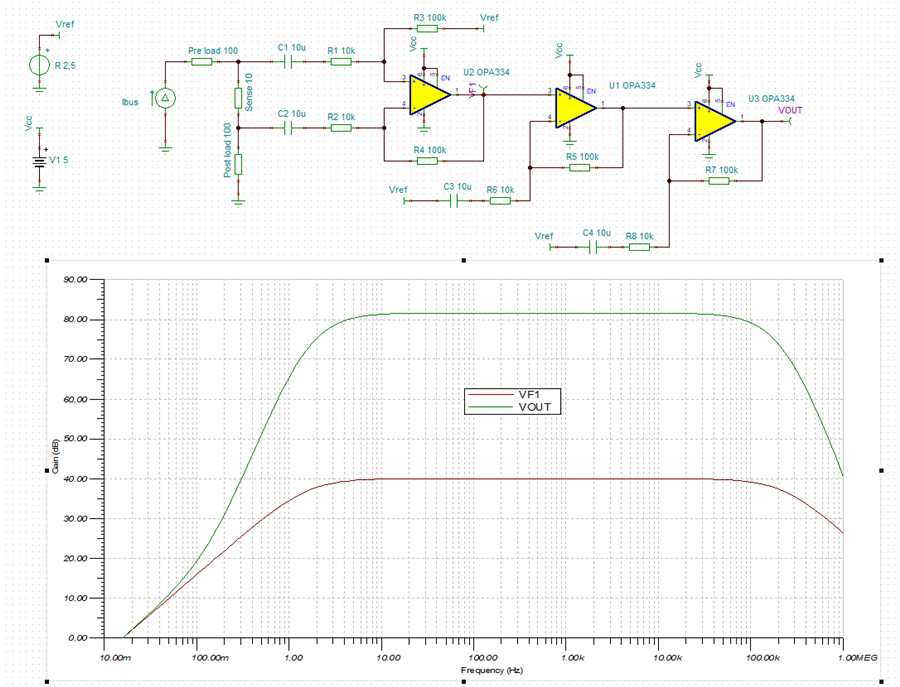
I built a current source using this circuit.
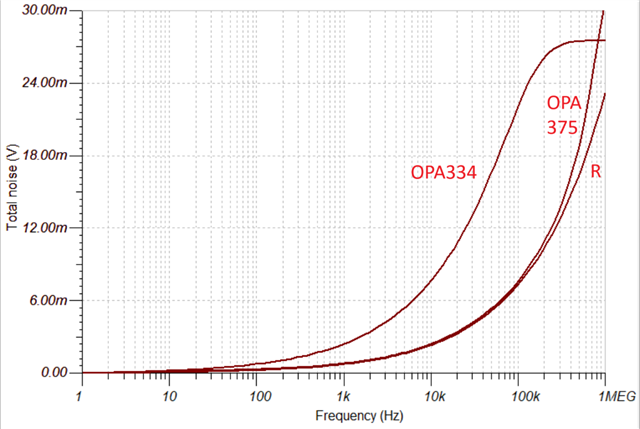
According to the simulation, the current noise is about 3 uArms ("total noise" in Tina-TI, analysis from 1 to 10 MHz) with a maximum current noise density of about 30 nA/RtHz ("output noise" in Tina-TI).
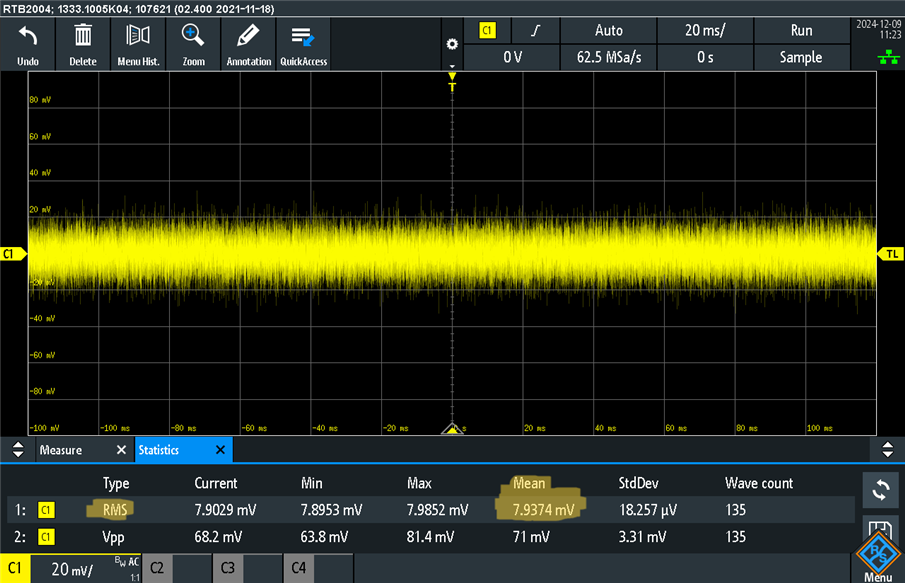
Now I want to validate the real circuit and measure the current noise of if.
How do I do this?
I cannot simply use a difference amplifier and a shunt as I want to measure the AC component and disregard the DC component of the current.
Further, I understand that I will need a spectrum analyzer to measure noise density.
To get started, it will be sufficient, though, to just measure the total RMS noise. Would a oscilloscope suffice for that measurement?
Thank you and have a good weekend!
Dan